An equilateral triangle is inscribed in a circle. A second circle is then inscribed in this triangle.
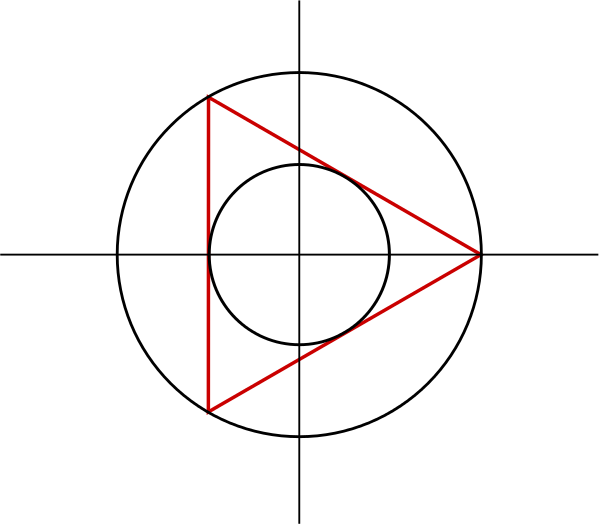
Can you create an image like this using Desmos or other graphing software?
(Note: In Desmos, to draw the line \(y=2x\) only between \((0,0)\) and \((2,4)\), you would type “\(y=2x \{0<x<2\}\)” or “\(y=2x \{0<y<4\}\)”.)
For each of the three outer circles given below, find the equation of the corresponding inner circle.
- \((x+2)^2+(y+1)^2=4\).
- \((x-4)^2+y^2=9\)
- \(x^2+y^2-3x-y=\frac{45}{2}\)
For each of the three inner circles given below, find the equation of the corresponding outer circle.
- \((x-3)^2+(y+\frac{1}{2})^2=16\)
- \((x+\frac{3}{2})^2+(y-\frac{3}{2})^2=8\)
- \(x^2+y^2+14y+29=0\)
What is the area of the equilateral triangle in terms of \(r\), the radius of the inner circle?
Another triangle…
If we now look at this similar image:
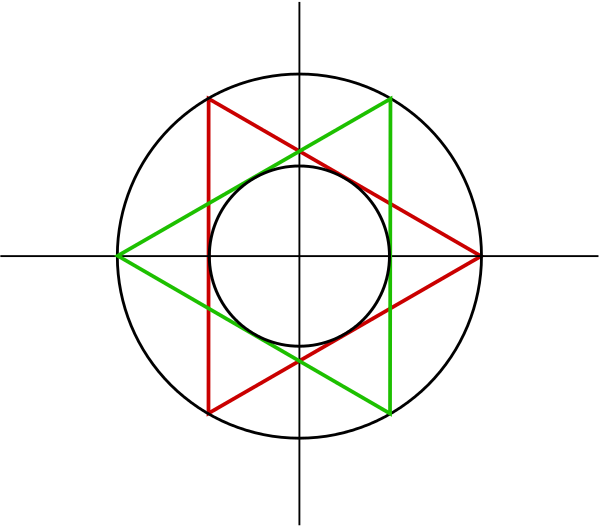
Can you create an image like this using Desmos or other graphing software?
Show that the ratio of the area of the original equilateral triangle to that of the enclosed hexagon is \(3:2\).
If you liked this problem you might like to have a go at Olympic rings.

